Compound interest has been famously described by Albert Einstein as ‘the most powerful force in the universe’. In a recent submission to the Productivity Commission1, the Association of Superannuation Funds of Australia (ASFA) likewise highlighted the virtues of compounding, indicating that investment returns will account for about 70% of the final value of an individual’s superannuation balance at retirement. ASFA’s assessment is based on an individual with average earnings who makes “contributions throughout a full working life”. Under this scenario, the remaining 30% of super saving by retirement age would be funded by the fund member’s own contributions.
The power of compound interest
Compounding interest is simply interest earned on not only your capital, but also on previously earned interest. To see how this works and how powerful it can be, let’s look at a simple example. In the table below we invest $1,000 into a managed fund earning 10% per annum. We can choose to either take the earnings each year or reinvest these by adding them to the account balance.
| Interest paid out | Interest compounded | |||
| Year | Investment Amount | Interest Paid Out | Investment Amount | Plus Interest Re-invested |
| 1 | $1,000 | $100 | $1,000 | $100 |
| 2 | $1,000 | $100 | $1,100 | $110 |
| 3 | $1,000 | $100 | $1,210 | $121 |
| 4 | $1,000 | $100 | $1,331 | $133 |
| 5 | $1,000 | $100 | $1,464 | $146 |
| 6 | $1,000 | $100 | $1,611 | $161 |
| 7 | $1,000 | $100 | $1,772 | $177 |
| 8 | $1,000 | $100 | $1,949 | $195 |
| 9 | $1,000 | $100 | $2,144 | $214 |
| 10 | $1,000 | $100 | $2,358 | $236 |
| Total interest earned* | $1,000 | $1,594 | ||
* For simplicity, the illustration has not taken into account taxation, volatility, and the investor’s cash flow needs.
While simplistic, the impact of compounding investment earnings is clear. When reinvested, the interest earned each year is added to the carry over account balance. Over ten years, the reinvestment strategy generated more than one and a half times the value of the pay-out option. If we extend the calculation over 30 years (below) our investor would be $13,449, or 548%, better off by compounding the returns.
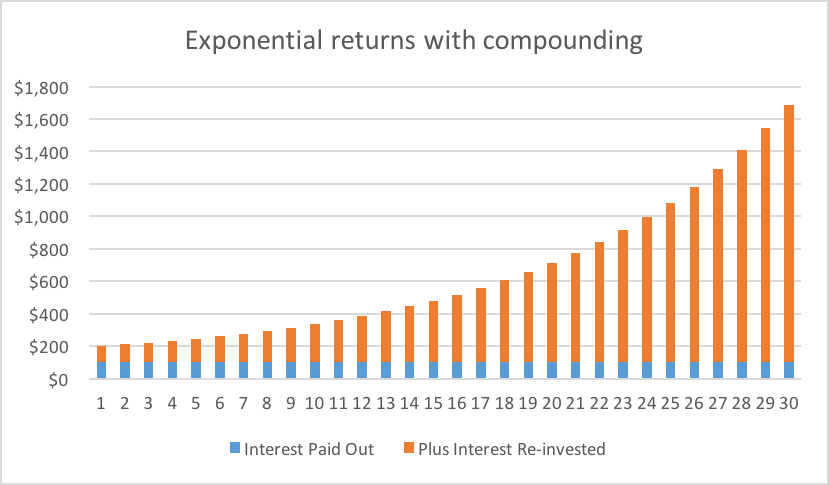
Pretty impressive! But what if you just want to work out how long it will take for your money to double while quietly compounding away?
Well, luckily there’s a handy little approximation technique known as the Rule of 72 which lets you quickly estimate this if you know the rate of return.
The rule of 72
Say you have an investment with an annual return of 8%. How long would it take for your initial investment to double in value? To estimate this, simply divide 72 by 8. And presto, the answer is 9. So, with an annual return of 8%, it will take approximately 9 years to double your initial investment.
Start now
So, regardless of the rate of return you aim to achieve on your investments, if you have time on your side the effect of compounding will provide a huge boost to the end result. Starting early and staying committed to regular saving is a proven way of creating long term wealth.
Now, all that’s a lot to take on board! So, why not ask your advisor how you can make the magic of compounding returns work best for you!
1. Quoted in the Productivity Commission draft report: How to Assess the Competitiveness and Efficiency of the Superannuation System.













